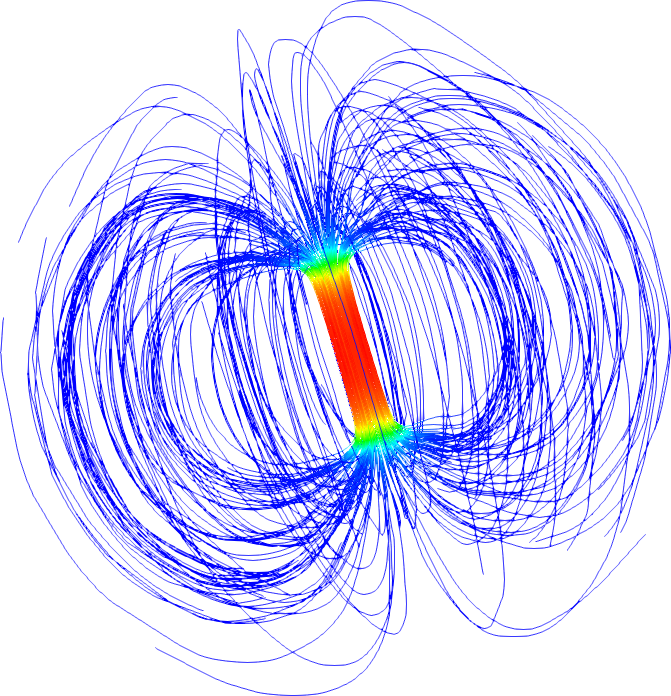
The use of electromagnetic fields is fundamental in engineering for the development of devices such as motors and transformers. The need to calculate magnetic field intensity (H) and magnetic flux density (B) becomes complex, as these devices often exhibit a three-dimensional field distribution. These problems are governed by Maxwell's electromagnetism laws, involving vector quantities that must be resolved at each point in the problem domain. Given that this domain generally has irregular and complex geometries, the analytical solution of the integro-differential equations is not always feasible.

Considering that H and B are functions of space and time, determined by parameters such as conductor geometry and the magnetic properties and structures of the materials (which are often nonlinear), the analysis involves both linear and nonlinear problems. Appropriate simplification assumptions often make it possible to reduce the electromagnetic field problem to the simpler concept of a circuit. The general three-dimensional problem can be approximated and reduced to a one-dimensional problem for analysis purposes.

This reduction results in a very useful simplification, where the three-dimensional field becomes a one-dimensional circuit, known as a magnetic circuit. In general, a magnetic circuit consists mostly of high-permeability magnetic flux material with a substantially uniform cross-section in which the magnetic flux is largely confined. Thus, magnetic circuit analysis reshapes the problem, simplifying the geometry and using scalar quantities instead of vector ones.